The surface area of a rectangular prism with sides a, b and c is given by the formula:
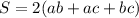
Use that formula to find the surface area that a prism with the given dimensions on each option would have.
A. 6, 2, 1 1/2
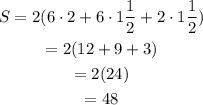
B. 5, 4, 1 1/4

C. 3, 4, 1 1/2
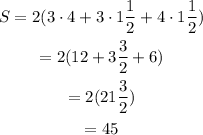
D. 6, 3, 1 1/3
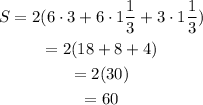
Therefore, the only possible dimensions of a rectangular prism with surface area 60 listed on the options, are 6, 3 and 3 1/3. The answer is: