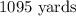Question

Campsite A and campsite B are located directlyopposite each other on the shores of Lake Omega,as shown in the diagram below. The two campsitesform a right triangle with Sam's position, S. Thedistance from campsite B to Sam's position is1,300 yards, and campsite A is 1,700 yards from hisposition1,700 yardsLake OmegaB1,300 yardsSWhat is the distance from campsite A to campsiteB, to the nearest yard?

Answer 1

The distance from campsite A to campsite B is;

`1095\text{ yards}`

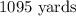
Step-by-step explanation:

Given that The two campsites A and B form a right triangle with Sam's position, S.

The distance from campsite B to Sam's position is 1,300 yards, and campsite A is 1,700 yards from his position;

`\begin{gathered} AS=1700\text{ yards} \\ BS=1300\text{ yards} \end{gathered}`

Applying pythagorean theorem, to solve for the third side AB;

`\begin{gathered} AS^2=AB^2+BS^2 \\ \text{making AB the subject of formula;} \\ AB^2=AS^2-BS^2 \\ AB=\sqrt[]{AS^2-BS^2} \end{gathered}`

Substituting the given values;

`\begin{gathered} AB=\sqrt[]{1700^2-1300^2} \\ AB=\sqrt[]{1200000} \\ AB=1095\text{ yards} \end{gathered}`

Therefore, the distance from campsite A to campsite B is;

`1095\text{ yards}`