Question

A software company has found that on Mondays, the polynomial function C(t) = −0.0625t4 + t3 − 6t2 + 16t approximates the number of callers to its hotline at any one time. Here, t represents the time, in hours, since the hotline opened at 8:00 A.M. How many service technicians should be on duty on Mondays at noon if the company doesn't want any callers to the hotline waiting to be helped by a technician?

Answer 1

At least 16 service technicians

Explanation:

Given

`C(t) = -0.0625t^4 + t^3 - 6t^2 + 16t`

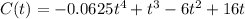
Required

Number of service technician to be online at 12 noon

First, we calculate the value of t

`t = 12\ Noon - 8:00AM`

`t = 4`

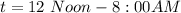
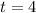
Substitute
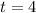`t = 4` in
`C(t) = -0.0625t^4 + t^3 - 6t^2 + 16t`

`C(4) = -0.0625 * 4^4 + 4^3 - 6 * 4^2 + 16 * 4`

`C(4) = 16`

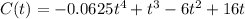
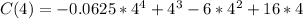
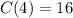
This implies that there are 16 customers online at 12 noon.

If 16 customers are online, then there should be at least 16 service technicians online.