Question

The diameter of a circle is 12 meters. What is the area of a sector bounded by a 102° arc?Give the exact answer in simplest form.

Answer 1

The area of the sector is;

`\begin{gathered} 10.2\pi m^2 \\ or \\ 32.04m^2 \end{gathered}`

Step-by-step explanation:

The Area of a sector can be calculated using the formula;

`A=(\theta)/(360)*\pi r^2`

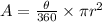
Where:

A = area of the sector

Angle theta = the angle bounding the sector

r = radius

Given:

`\begin{gathered} \theta=102^0 \\ r=\frac{\text{diameter}}{\text{2}}=(12m)/(2)=6m \\ r=6m \end{gathered}`

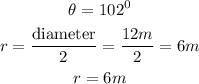
substituting the given values, we have;

`\begin{gathered} A=(102)/(360)*\pi(6^2) \\ A=10.2\pi m^2 \\ A=32.04m^2 \end{gathered}`

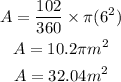
Therefore, the area of the sector is;

`\begin{gathered} 10.2\pi m^2 \\ or \\ 32.04m^2 \end{gathered}`