a)
Using conservation of energy for ball 1:
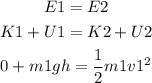
Solve for v1:
![\begin{gathered} v1=\sqrt[]{2gh} \\ v1=\sqrt[]{2(9.8)(2.5)} \\ v1=7(m)/(s) \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/mvifeq30t5idhsjmlwbt4e2qubnic8czyb.png)
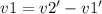
Using conservation of momentum:
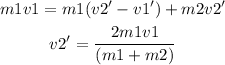
a)
m2 = 5 kg
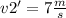
so:
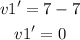
so:
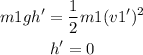
If the stationary mass is 5.0 kg the height back up the ramp is 0 meters
b)
m2 = 10

so:
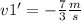

If the stationary mass is 10.0 kg the height back up the ramp is 0.2778 meters
c)
m2 = 500.00kg
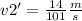
so:
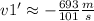
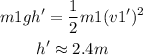
If the stationary mass is 500.0 kg the height back up the ramp is 2.4 meters