Given:
The given function is:
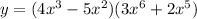
First method: Multiplying factors and differentiating after arranging like terms together.

Now we will differentiate y with respect to x by basic rules:
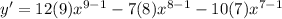
Solving further,
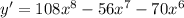
(b) Second method: Apply product rule to find the derivative:
Again,
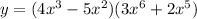
The product rule states:
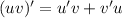
Where u and v are the two factors multiplied.
So here we have:
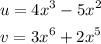
Finding the derivatives:
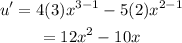
Similarly,

Now put the values in the product rule,
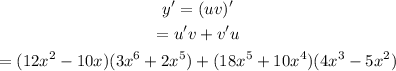
Simplifying further,
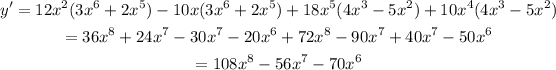
This is the derivative obtained.
From above two methods, we can see the derivative is same in both the cases.