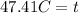The idea behind the problem is solving an equation involving square roots. The equation I'm talking about is
![358=20\cdot\sqrt[]{273+t}](https://img.qammunity.org/2023/formulas/mathematics/college/ts8aoa4gkabujvu186o7bui6zhxueqirot.png)
(I merely replaced v by 358; what we are supposed to do is to find t). Let's solve it:
1. 20 is multiplying at the right-hand side, let's send it to divide at the left:
![(358)/(20)=\sqrt[]{273+t}](https://img.qammunity.org/2023/formulas/mathematics/college/xw7zjlh0afs0jabc7u8zciwqd713tq1i6z.png)
2. (this is the most important step) Take the power of 2 on both sides:
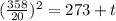
...........................................................................................................................................................
Comment: Remember that
![(\sqrt[]{273+t})^2=273+t](https://img.qammunity.org/2023/formulas/mathematics/college/lyxq0bbkftoovnq409qn53ah3l2mximooe.png)
because square root and powering by two are inverse of each other.
...........................................................................................................................................................
3. Put the left-hand side in a calculator to get:
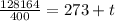
4. Let's subtract 273 to the left-hand side: