Given:
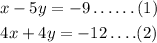
The given point is (-4,1)
Let's check the ordered pair (-4,1) in the first equation
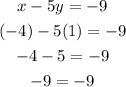
Hence, (-4,1) is a solution of the first equation x-5y=-9.
Now, let's check (-4,1) in the second equation.
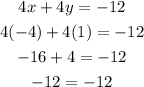
So, (-4,1) is a solution of the second equation 4x+4y=-12.
Hence, (-4,1) is a solution of both equation in the system, then it is a solution to the overall system.