We are given the function below;
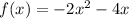
PART A
We then proceed to find if the function has a minimum or maximum value. To find if the function has a minimum or maximum value. If the x^2 coefficient is positive, the function has a minimum. If it is negative, the function has a maximum.
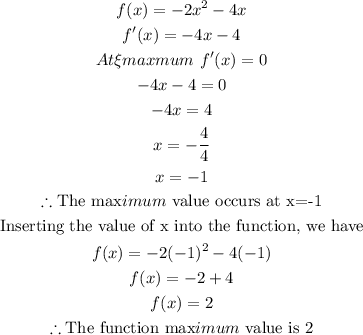
ANSWER: From the above, we can see that x^2 is negative, hence the function has a maximum
PART B and C
To find the minimum or maximum value, we would plot the graph of the f(x). The graph can be seen below.
From the graph, the black point helps answer part A and part B.
ANSWER: The function's maximum value is f(x)=2.
This is the point where the slope of the graph is equal to zero
ANSWER: The maximum value then occurs at x= -1
We can also solve this by differentiating the function.