Question

A parabola has a vertex at (2, -1) and a y- intercept at (0,3). Is this enough information to sketch a graph? Explain your answer. Henny Yoffe . 11:20 AM

Answer 1

It is given that the parabola has the vertex at (2,-1)and y intercept of 3,

Consider the general equation of the parabola with vertex (p,q),

`y=a(x-p)^2+q`

Sbstitute 2 for 'p' and -1 for 'q',

`y=a(x-2)^2-1`

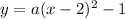
Given that the y-intercept is 3, it means that the curve passess through (0,3),

So it must satisfy the equation,

`3=a(0-2)^2-1\Rightarrow4a=4\Rightarrow a=1`

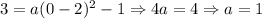
Substitute the value of 'a', 'p', and 'q' in the standard equation,

`y=1(x-2)^2-1\Rightarrow y=(x-2)^2-1`

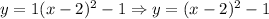
Thus, the equation of the parabola can be obtained using the given conditions.