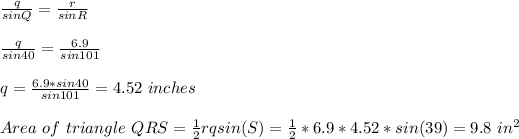Question

In ΔQRS, r = 6.9 inches, ∠Q=40° and ∠R=101°. Find the area of ΔQRS, to the nearest 10th of an square inch.

Answer 1

9.8 in²

Explanation:

If A, B and C are the angles of a triangle and a, b, c are the repective sides opposite the angles, then the area of the triangle is given as:

Area = (1/2)absin(C)

In ΔQRS, the area is:

Area = (1/2)rqsin(S)

∠S + ∠Q + ∠R = 180° (sum of angles in a triangle)

∠S + 40 + 101 = 180

∠S = 39°

Using sine rule to find q:

`(q)/(sinQ) =(r)/(sinR) \\\\(q)/(sin40)=(6.9)/(sin101) \\\\q=(6.9*sin40)/(sin101) =4.52 \ inches\\\\Area\ of\ triangle \ QRS=(1)/(2)rqsin(S) =(1)/(2)*6.9*4.52*sin(39)=9.8\ in^2`