We have to find the equation of the line that passes through points (2,-5) and (7,3).
We can start by calculating the slope m as:
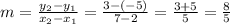
With one point and the slope, we can write the line equation in slope-point form and then rearrange it:
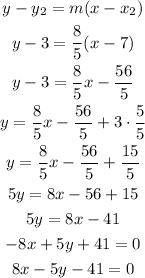
The equation in general form is 8x-5y-41 = 0.
We can sketch it as: