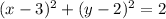Question

The center of a circle and a point on the circle are given. Writecenter: (3,2), point on the circle: (4,3)

Answer 1

Given:

The center of the circle is the point ( 3, 2 )

And the point on the circle is ( 4, 3 )

To write the equation of the circle, we need to find the radius

The radius = the distance between the center and the point on the circle

so, the radius is the distance between ( 3, 2) and ( 4, 3)

So,

`\begin{gathered} r=d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2} \\ r=\sqrt[]{(4-3)^2+(3-2)^2^{}}=\sqrt[]{1+1}=\sqrt[]{2} \end{gathered}`

The general equation of the circle is:

`(x-h)^2+(y-k)^2=r^2`

Where (h, k) is the coordinates of the center of the circle, r is the radius

So,

`\begin{gathered} (h,k)=(3,2) \\ r=\sqrt[]{2} \end{gathered}`

so, the equation of the circle will be:

`(x-3)^2+(y-2)^2=2`