Your brother and sister took turns driving on a 635 mile trip
Total distance travel on trip = 635
Let x be the disatnce travel by your borther and y be the miles travel by the sister
SO, x + y = 635
It took 11 hours to complete the trip
Speed of brother = 60 miles per hour
Speed of sister = 55 miles per hour
The general expression for the speed is :
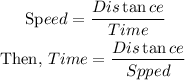
Then using these expression
Time taken by the brother is :
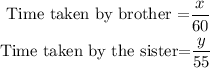
As total time is 11 hours so:
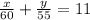
So we get the two set of equation :
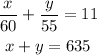
Simplify the set of equation :
Simplify the first equation for x and then put it into another :
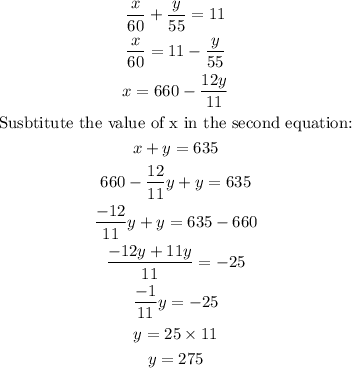
Substitute y = 275 in the first equation :
x + y = 635
x + 275 = 635
x = 360
As x represent the distance travel by the bother and the rest by sister
Distance travel by brother is 360 miles and the distance travel by the sister is 275 miles
Answer : x = 360 miles, y = 275 miles