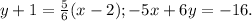Recall that the equation of a line that passes through two points is given by the following formula:
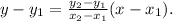
Notice that the above formula gives us the equation of the line in point-slope form. Substituting the given values in the above formula, we get:
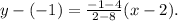
Simplifying the above result, we get:
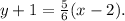
Now, taking the above equation to its standard form, we get:
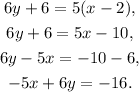
Answer: