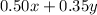Answer:
The expression for the amount of money in dollars he could earn is;
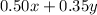
Step-by-step explanation:
Let x represent the number of cups of large cups of lemonade he sell and
y represent the number of cups of the small cups of lemonade he sell.
Given that;
He sells large cups of lemonade for $0.50
and small cups of lemonade for $0.35.
The amount he will male by selling x cups of large cups of lemonade and y cups of small cups of lemonade is;
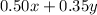
The expression for the amount of money in dollars he could earn is;