Given:
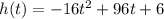
Find-:
(a) Maximum second after launch will the object reach its maximum height
(b) Find the maximum height that the object reaches.
(c) Find the x-intercept and explain its meaning in the context of the problem.
(d) After how many seconds will the object be 100 feet above the ground
(e) Find the y-intercept and explain its meaning on the context of the problem
Sol:
(a)
Maximum second after launch.
For maximum value derivative should be zero.
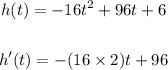

After 3-second the object reaches maximum height.
(b)
For maximum height is at t = 3
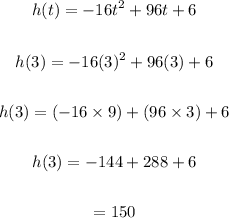
(c) x-intercept the value of y is zero that means:

The negative value of "t" is not considered so at
x-intercept is 6 and -0.061
(d) Object be 100 feet above grounded is:

So, the time is:
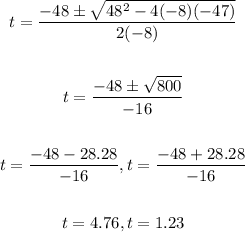
At t= 4.76 and t =1.23
(e)
For y-intercept value of "x" is zero.
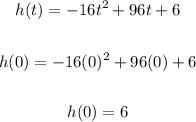
So, the y-intercept is 6.