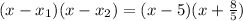Question

Factor the quadratic expression.5x2 – 17x - 405x2 - 17x - 40 = (Factor completely.)

Answer 1

The general form of a quadratic polynomial is given by:

`ax^2+bx+c`

You have the following quadratic expression:

`5x^2-17x-40`

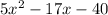
In order to factorize the previous expression, you first use the quadratic formula, which is given by;

`x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`

where a = 5, b = -17, c = -40. You replace these values into the quadratic formula:

`\begin{gathered} x=\frac{-(-17)\pm\sqrt[]{(-17)^2-4(5)(-40)}}{2(5)} \\ x=\frac{17\pm\sqrt[]{1089}}{10}=(17\pm33)/(10) \\ x_1=5 \\ x_2=\text{ -}(16)/(10)=-(8)/(5) \end{gathered}`

The factors of the quadratic polynomial, based on the previous calculated zeros of the piolynomial are as follow:

`(x-x_1)(x-x_2)=(x-5)(x+(8)/(5))`