Question

A student was asked to find the length of the unknown leg of the right triangle. He incorrectly said that the length of the unknown leg of the right triangle is about 6.2 cm. Find the length of the unknown leg of the right triangle.The length of the unknown leg of the triangle is ______cm. (Round to one decimal place as needed.)What mistake might the student have made? A. He added the two given valuesB. He subtracted the two given values C. He did not square the length of the given leg D. He did not square the length of the hypotenuse

Answer 1

6 cm, C. He did not square the length of the given leg

Step-by-step explanation

to solve this we need to use the Pythagorean theorem

T.P states for all rigth triangles:

`a^2+b^2=c^2`

then

Step 1

let

a=2.3

b=b

c=6.4

replace

`\begin{gathered} 2.3^2+b^2=6.4^2 \\ \text{now, we n}eed\text{ isolate b} \\ 5.29+b^2=40.96 \\ \text{subtract 5.29 in both sides} \\ 5.29+b^2-5.29=40.96-5.29 \\ b^2=35.67 \\ \text{square root in both sides} \\ \sqrt[]{b^2}=\sqrt[]{35.67} \\ b=5.97 \\ \text{rounded} \\ b=6 \end{gathered}`

Step 2

What mistake might the student have made?

check

A)He added the two given values

`2.3+6.4=8.7`

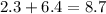
B) He subtracted the two given values

`6.4-2.3=4.1`

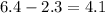
C)He did not square the length of the given leg

`\begin{gathered} 2.3^{}+b^2=6.4^2 \\ b^2=6.4^2-2.3 \\ b^2=38.66 \\ b=\sqrt[]{38.66} \\ b=6.21 \\ \text{rounded} \\ b=6.2 \end{gathered}`

D. He did not square the length of the hypotenuse​

`\begin{gathered} 2.3^2+b^2^{}=6.4 \\ b^2=6.4-2.3^2 \\ b^2=1.11 \\ b=\sqrt[]{1.11} \\ b=1.05 \end{gathered}`

so, the answer is C

I hope this helps you