Question

The probability that VSU and KSU both win a basketball game in the same week is 47%. Theprobability that VSU wins is 50%. What is the probability that KSU will win given that VSUhas already won?

Answer 1

Step-by-step explanation

Let the probability that KSU will win a game be Pr(A), and let the probability that VSU wins be Pr(B). Therefore;

`\begin{gathered} Pr(A\cap B)=0.47 \\ Pr(B)=0.5 \end{gathered}`

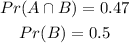
We can then use the conditional probability formula below.

`Pr(A|B)=(Pr(A\cap B))/(Pr(B))`

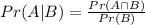
Therefore, the probability that KSU will win given that VSU has already won becomes

`Pr(A|B)=(0.47)/(0.50)=0.94`

Answer: 0.94