Answer:
Concept:
To figure out the length of the running track, we will use the following steps below
Step 1:
Calculate the length of the round the two semicircles
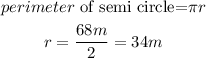
By substituting the values in the formula above, we will have

Step 2:
The image below will be used to calculate the length round the training track
Hence,
To calculate the length of the track we will have
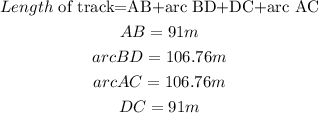
By substituting the values, we will have
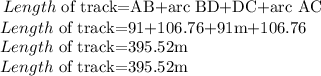
Hence,
The final answer = 395.52m