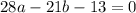We are given the equation of a curve;
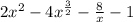
To solve this we begin by taking the derivative of this curve. Note that the slope of this curve is its first derivative.
We now have;
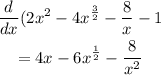
At this point we should note that the slope (gradient) is the value of this first derivative when x = 4.
We can now plug in this value and we'll have;
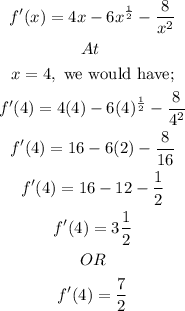
Now we can see the slope of the curve. The slope of the normal line perpendicular to the tangent of the curve is a negative inverse of this.
The negative inverse of 7/2 would be;
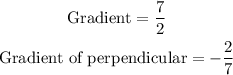
Now to use this value to derive the equation in the form
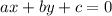
We start by expresing this in the form;

We now have;
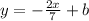
We can convert this to the standard form as indicated earlier;

With the points
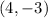
We now have, the equation;
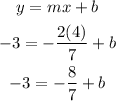
We now collect like terms;

We now have the y-intercept as calculated above.
We can now write up our equation is the standard form as indicated from the beginning;
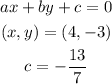
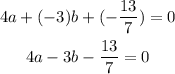
Note that A, B and C must be integers. Therefore, we multiply all through by 7;
ANSWER: