Question

CJ loves Girl Scout cookies. He eats 3 cookies per hour. After 5 hours, there are 24 cookies left in the box. Write an equation in slope intercept form. Determine how many hours it will take CJ to eat the entire box of cookies.

Answer 1

To solve this problem: y will represent the number of cookies, and x the number of hours.

To find the number of cookies that CJ eats per hour, we multiply 3 (since he eats 3 per hour) by x (the number of hours)

Since there we only 24 cookies left in the box, we will need to substract 3 by the number of hours that have passed, from 24 to find the number of cookies "y":

`y=24-3(x-5)`

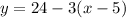
This equation represents that the number of cookies "y" is equal to the 24 cookies that where left after 5 hours, and to that we substract 3 (which is the number of cookies per hour) by total number of hours that have passed since those 5 hours (x-5) because 5 hours that have already passed we substract them from x.

We need to simplify that equation to represent in slope-intercept form:

`\begin{gathered} y=24-3x+15 \\ y=-3x+39 \end{gathered}`

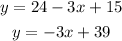
Now we need to determine the number of hours it would take to finish the cookies. So we are looking for the value of x, that makes y=0:

`0=-3x+39`

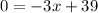
solving for the number of hours x:

`\begin{gathered} -3x=-39 \\ x=-(39)/((-3)) \\ x=13 \end{gathered}`

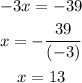
It would take 13 hours for CJ to eat the entire box of cookies.