As per given by the question.
There are given that a triangle.
Now,
Suppose given triangle is ABC.
Then, redraw the given triangle.
Now,
For finding the value of x, and y;
First find the value of x with the help of sine trigonometric function.
So,
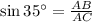
Then,
Substitute the value x for AB and 22 for AC.
SO,
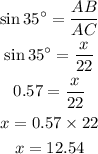
Now,
For finding the value of y,
Here, use pythagoras theorem in triangle ABC.
So, from the pythagoras theorem;
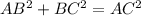
Then,
Substitute the value in above formula;
So,
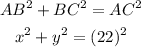
Now, put the value 12.54 for x,
So,

Then,
![\begin{gathered} y^2=326.75 \\ y=\sqrt[]{326.75} \\ y=18.076 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/35pd7rrvtirh3862tv9x213f18517d37ll.png)
Hence, the value of x is 12.54 and the value of y is 18.076.