There are 8 total outcomes. Now, we need to find where P(<7).
This means the outcomes where the number is less than 7.
There are 6 numbers under the 7 = 1,2,3,4,5,6
The probability is given by the next formula:
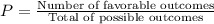
Where:
Number of favorable outcomes = 6
Total of possible outcomes = 8
Replacing these values:

Simplify the expression, divide both numbers by 2:
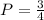
The correct answer is the second one.