Question

There are 38 coins in a collection of 20 paise coins and 25 paise coins. If the total value of the collection is Rupees 8.50, how many of each are there?

Answer 1

We will have the following:

*First:

**We stablish that x will represent the number of 20 paise coins.

**We stablish that y will represent the number of 25 paise coins.

Second: From this we will then have:

`x+y=38`

&

`20x+25y=850`

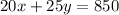
[This 850 is due to the fact that 8.50 Rupees are equal to 850 paise].

*Third: We solve for either x or y in the first equation:

`x=38-y`

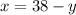
Now, we replace this in the second equation and solve for y:

`20(38-y)+25y=850\Rightarrow760-20y+25y=850`
`\Rightarrow5y=90\Rightarrow y=18`

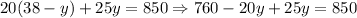
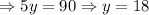
So, we have that there are 18 25 paise coins.

Now, using this we solve for x in the first equation:

`x+18=38\Rightarrow x=20`

So, we have that there are 20 20 pais coins.