1) Considering that the 1st property of Logarithms tells us:
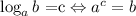
2) Let's evaluate that:
![\begin{gathered} \log _{\csc ((5\pi)/(6))}((\csc \pi)/(4))=x\Rightarrow\csc ((5\pi)/(6))^x=\csc ((\pi)/(4)) \\ \\ \csc ((5\pi)/(6))^x=\csc ((\pi)/(4)) \\ \log \csc ((5\pi)/(6))^x=\log \csc ((\pi)/(4)) \\ \text{x}\log \csc ((5\pi)/(6))^{}=\log (\sqrt[]{2}) \\ x\log (2)=\text{ log(}\sqrt[]{2}) \\ x=\frac{\log\sqrt[]{2}}{\log\text{ 2}} \\ x=(1)/(2) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/64uwp7ehat8fgk8lyzfegpljzygvz3ar9c.png)
• Notice that we've descended that exponent x, to become a factor (3rd line).
,
• Then divided both log with a common base, in this case, base 10.
3) Hence that equation yields 1/2 as its result.