Question

Put the steps in order to find the distance between these

Answer 1

1., 2., 4., 5., 6., 3., 7.

Explanation:

1. Draw a right triangle by dropping a vertical side and a horizontal side. (See attachment).

2. Determine the vertical side (2 units) and horizontal side (6 units) lengths by counting on the grid (be careful of the scale), or using the vertical coordinated (3 to 1) and horizontal coordinate (-2 to 4).

`\boxed{\begin{minipage}{9 cm}\underline{Pythagoras Theorem} \\\\$a^2+b^2=c^2$\\\\where:\\ \phantom{ww}$\bullet$ $a$ and $b$ are the legs of the right triangle. \\ \phantom{ww}$\bullet$ $c$ is the hypotenuse (longest side) of the right triangle.\\\end{minipage}}`

Therefore:

• a = 2
• b = 6

4. Use the Pythagorean Theorem for right triangles to determine the diagonal length:

`\implies \sf 2^2+6^2=c^2`

`\sf 5.\quad 4 + 36 = c^2`

`\sf 6. \quad 40 = c^2`

`\sf 3.\quad √(40)=√(c^2)`

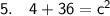
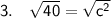
7. √40 is between √36 and √49, so between 6 and 7 - closer to 6, so about 6.3 units.

Answer 2

• See attached

Explanation:

The distance between the points is found using the given coordinates and the distance formula.

The distance formula is based on right triangle and Pythagorean theorem and is the calculation of the hypotenuse provided the legs are known.

So the right order of operations is attached for you below.

You have found most of it, just small correction is needed.