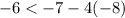The Solution:
Given:
Required:
Find f(2):
![\begin{gathered} f(2)=√([(-5*2)+14])=√(-10+14)=√(4)=2 \\ \\ f(2)=2 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/4ce1shrmk1mwx7guyu2q8vyepgm22d1uej.png)
Find g(-5):
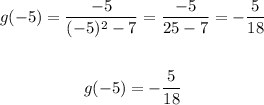
Find h(-1/2):
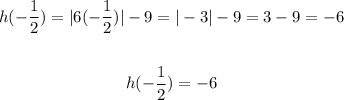
Answer:
f(2) =
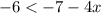
now we can solve the inequalty for x by passing the -7 to the other side:
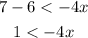
Now to change the sign of the -4x we have to invert the inequality:

so the only solution is -8 and we can prove it: