Given:
The sum of the perimeters of similar quadrilaterals is 22.5 dm. The similarity coefficient k = 1.25.
Required:
To find the perimeters of the quadrilaterals.
Step-by-step explanation:
The quadrilaterals are similar and the similarity coefficient is k = 1.25.
If the quadrilateral is similar then the ratio of their corresponding sides is equal.
Let the perimeter of the one quadrilateral is x then the perimeter of the other quadrilateral will be 1.25x
Now
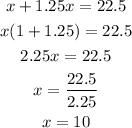
Thus the perimeter of the one quadrilateral is 10dm.
The perimeter of the other quadrilateral = 1.25 x 10 = 12.5 dm
Final Answer:
Thus the perimeter of the quadrilaterals is 10dm and 12.5 dm.