In order to calculate the sector area, we can use the following rule of three, knowing that an angle of 2pi (complete circle) has an area of pi*r² (area of the circle).
So we have:
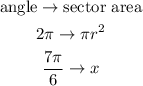
Now, we can write the following proportion and solve the equation for x:
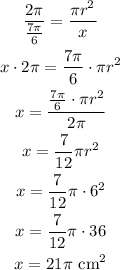
Therefore the sector area is 21pi cm².