We could state an exponential model.
Number of bateria will be given by:
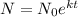
where k=growth constant and t=time in minutes.
We're given that N0 is the initial population, which is:
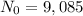
We also know that, when t=236 min, N=2(9,085) = 18,170:
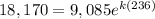
We're going to solve this equation to find the value of k:
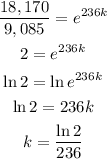
Then, our expression is:
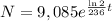
To find the population of bacteria after 708 minutes, we replace t by 708:

Therefore, the population of bacteria 708 minutes from now, is 72680.