Question

Solve the right triangle ABC for all missing parts. Express all angles in decimal degrees. a= 306.5 km, c=591.3 km(Round to the nearest hundredth as needed)

Answer 1

`\begin{gathered} b=505.66\text{ km} \\ A=37.56\~ \\ B=58.77\text{ \degree} \\ C=90\text{ \degree} \end{gathered}`

Step-by-step explanation

Step 1

we have a rigth triangle, then

let

`\begin{gathered} side_1=306.5 \\ side_2=b \\ \text{hypotenuse}=591.3 \end{gathered}`

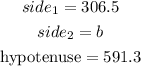
to find the missing side we an use the Pythagorean theorem. it states that the sum of the squares on the legs of a right triangle is equal to the square on the hypotenuse (the side opposite the right angle)

so

`\begin{gathered} side^2+side^2=hypotenuse^2 \\ \text{replace} \\ 306.5^2+b^2=591.3^2 \\ \text{subtract }306.5^2i\text{n both sides} \\ 306.5^2+b^2-306.5^2=591.3^2-306.5^2 \\ b^2=591.3^2-306.5^2 \\ b=\sqrt[]{591.3^2-306.5^2} \\ b=\sqrt[]{255693.44} \\ b=505.66\text{ km} \end{gathered}`

hence

b=505.66 km

Step 2

angles

a)A

and B

`\begin{gathered} \sin \text{ B=}(b)/(c) \\ B=\sin ^(-1)((505.66)/(591.3)) \\ B=58.77\text{ \degree} \end{gathered}`

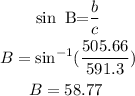
I hope this helps you