Question

In the parallelogram below, if AP = 45 and PC = 3x + 15, find x.

Answer 1

One of the properties of a parallelogram is that its diagonals bisect each other. This means that one diagonal cuts the second diagonal in equal length.

This means that AP = PC. If AP = PC, then 45 = 3x + 15.

`45=3x+15`

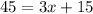
We can now find the value of x using the equation above.

First, subtract 15 on both sides of the equation.

`45-15=3x+15-15`
`30=3x`

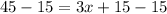
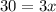
Then, divide both sides of the equation by 3 to isolate x.

`(30)/(3)=(3x)/(3)`
`10=x`

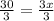
Therefore, the value of x is 10. (Option B)