Solution
(a). For any number of equations to be solved simultaneously, the number of equations, must be same as number of variables.
Hence, Equation (1) & (2) can't be solved simultaneously, because, only two equations are given to solve for 3 variables.
(b) From the explanation above, it is obvious that, Equation (1), (2), and (3), can be solved simultaneously, because, we have 3 variables (x, y, z), with 3 equations to solve with.
Next we do is to solve Equation (1), (2), and (3) simultaneously using substitution method.

From the Equation 2, make y the subject of formula
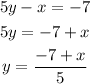
We substitute, for y in equation (1), and (3).
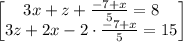
Simplifying,
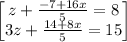
make z the subject of formula
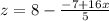
Substitute z in the second equation,
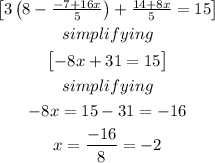
Now, we have the value of x, remaining y, and z, and we substitute the value of x = -2, in the equation above for z.
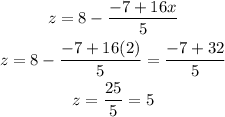
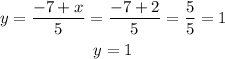
Hence, x = -2, y = 1, z = 5
(c)
Next, we proof for the values of x, y, and z in equation (4)
Substitute, x = -2, y = 1, z = 5 in equation (4)

Hence, the solution doesn't hold for the equation (4).