We are to maintain a constant mean distance of ( d-avg ) to qualify for the cross-country biking expedition.
The qualification for the expedition is to rirde an average distance of:
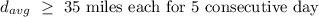
We are already on target for 4 days. For which we covered a distance ( d ) for each day:

We are to project how much distance we must cover atleast on the fifth day ( Day 5 ) so that we can qualify for the expedition. The only condition for qualifying is given in terms of mean distance traveled over 5 days.
The mean value of the distance travelled over ( N ) days is expressed mathematically as follows:
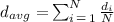
Where,
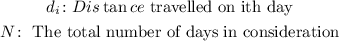
We have the data available for the distance travelled for each day ( di ) and the total number of days in consideration ( N = 5 days ). We will go ahead and used the standard mean formula:
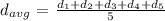
Then we will apply the qualifying condtion to cover atleast 35 miles for each day for the course of 5 days.
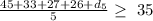
Then we will solve the above inequality for Day 5 - (d5) as follows:
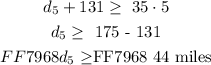
The result of the above manipulation shows that we must cover a distance of 44 miles on the 5th day so we can qualify for the expedition! So the range of distances that we should cover atleast to qualify is:

All covered distances greater than or equal to 44 miles will get us qualified for the competition!