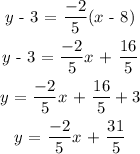Question

Please I need help finding the equation of the parallel line and the perpendicular line.

Answer 1

Answer:

The equation parallel to the given equation and passing through the point (8, 3) is:

`y\text{ = }(5)/(2)x\text{ - 17}`

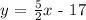
The equation perpendicular to the given equation and passing through the point (8, 3) is:

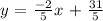
`y\text{ = }(-2)/(5)x\text{ + }(31)/(5)`Explanations:

The equation of the line parallel to the line y = mx + c and passing through the point (x₁, y₁) is given as:

`y-y_1=m(x-x_1)`

The equation of the line perpendicular to the line y = mx + c and passing through the point (x₁, y₁) is given as:

`y-y_1\text{ = }(-1)/(m)(x-x_1)`

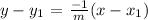
Now, for the equation:

`\begin{gathered} y\text{ = }(5)/(2)x\text{ - 7} \\ m\text{ = }(5)/(2) \end{gathered}`

The line parallel to the equation and passing through the point (8, 3) will be:

`\begin{gathered} y\text{ - 3 = }(5)/(2)(x\text{ - 8)} \\ y\text{ - 3 = }(5)/(2)x\text{ - 20} \\ y\text{ = }(5)/(2)x\text{ - 20 + 3} \\ y\text{ = }(5)/(2)x\text{ - 17} \end{gathered}`

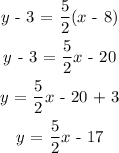
The line perpendicular to the given equation and passing through the point (8, 3) will be:

`\begin{gathered} y\text{ - 3 = }(-2)/(5)(x\text{ - 8)} \\ y\text{ - 3 = }(-2)/(5)x\text{ + }(16)/(5) \\ y\text{ = }(-2)/(5)x\text{ + }(16)/(5)+3 \\ y\text{ = }(-2)/(5)x\text{ + }(31)/(5) \end{gathered}`