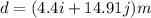Question

A hiker walks 14.91 m, N and 4.40 m, E. What is the magnitude of his resultant displacement?

Answer 1

Givens.

• 14.91 meters North.

,

• 4.40 meters East.

First, make a diagram to visualize the vectors and the resultant displacement.

In the figure, the purple vector d represents the resultant displacement, which horizontal component is 4.40m and its vertical component is 14.91m.

Let's use the following formula to find the resultant.

`d=\sqrt[]{(y_{})^2+(x)^2}`

Where y = 14.91 and x = 4.40.

`\begin{gathered} d=\sqrt[]{(14.91m)^2_{}+(4.40m)^2} \\ d=\sqrt[]{222.31m^2+19.36m^2} \\ d=\sqrt[]{241.67m^2} \\ d\approx15.55m \end{gathered}`

Therefore, the magnitude of the resultant displacement is 15.55m.

But, the resultant displacement refers to the vector, which is the following

`d=(4.4i+14.91j)m`