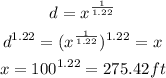The expression to calculate the distance a person can see is below, where x is the height in feet of a person's eyes above see-level:
![d=\sqrt[1.22]{x}\lbrack mi\rbrack](https://img.qammunity.org/2023/formulas/mathematics/college/smbheclzs33sk5y27szskta58lxmm4fwgf.png)
a) A person who is 600 feet height will see:
![d=\sqrt[1.22]{600}=189.30mi](https://img.qammunity.org/2023/formulas/mathematics/college/f2oz1ndenb7ljumpsvi4c7dwjbkhe4e151.png)
b) In order to get the height a person needs to be so that he/she could see 100 miles long, we solve the equation for x: