Question

A set of data items normally distributed with a mean of 60. Convert the data item to a z-score, if the data item is 47 and standard deviation is 13.

Answer 1

To answer this question, we need to remember what the z-score is. The formula for it is as follows:

`z_{\text{scorre}}=(x-\mu)/(\sigma)`

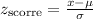
We have that:

• mu is the population mean

,

• x is the raw score we want to normalize or convert into a z-score

,

• sigma is the population standard deviation.

Then, since we have that the mean is equal to 60, the raw score, x, is equal to 47, and the standard deviation is 13, then, we have that the z-score is:

`z_{\text{score}}=(47-60)/(13)=(-13)/(13)\Rightarrow z_(score)=-1`

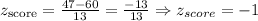
Then, the z-score is equal to -1. That is, x is one standard deviation below the population mean.