right
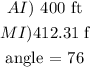
Step-by-step explanation
Step 1
AI?
we have a rigth triangle
then
let
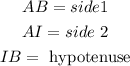
we can use the pythagorean Thoerem to find the missing vale
so
![\begin{gathered} (AB)^2+(AI)^2=(BI)^2 \\ \text{replace} \\ 300^2+(AI)^2=500^2 \\ so \\ (AI)^2=500^2-300^2 \\ AI=\sqrt[]{500^2-300^2}=\sqrt[]{160000}=400 \\ AI=400 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/yi36s10dqikjdaykgijw5a4970ibjyy8r6.png)
Step 2
MI?
let
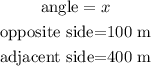
so, we need a function that relates those 3 values
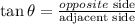
replace

As 76 is greater than 68, the zipline cable compliance with these regulations.
Also, the hypotenuse (zipline ) is
![\begin{gathered} (MI)^2=(AI)^2+(AM)^2 \\ \text{replace} \\ (MI)^2=(400)^2+(100)^2 \\ (MI)^2=170000 \\ MI=\sqrt[]{17000} \\ MI=412.31\text{ ft} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/x9qa6v89fsbs152ffp0fimabdh2b9n53co.png)
I hope this helps you