Question

You invested $5000 between two accounts paying 7% and 8% annual interest. If the total interest earned for the year was $380, how much was invested at each rate?

Answer 1

Let x be the amount invested in the account paying 7% and y the amount invested in the account paying 8%, then we can set the following system of equations:

`\begin{gathered} x+y=5000 \\ 0.07x+0.08y=380 \end{gathered}`

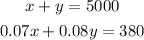
Solving the first equation for x and substituting it in the second equation we get:

`0.07(5000-y)+0.08y=380`

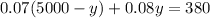
Solving for y we get:

`\begin{gathered} 350-0.07y+0.08y=380 \\ 0.01y=30 \\ y=3000 \end{gathered}`

Substituting y=3000 in the first equation and solving for x we get:

`\begin{gathered} x+3000=5000 \\ x=2000 \end{gathered}`

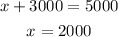
Therefore, $2000 was invested in the account paying 7%, and $3000 was invested in the account paying 8%.