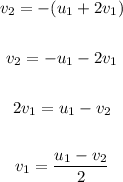Answer:
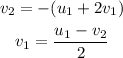
Step-by-step explanation:
Mass of object 1 = m₁
Velocity of object 1, = u₁
The mass of object 2 is half that of object 1
m₂ = m₁/2
The velocity of object 2 is three times the speed of object 1 but in the opposite direction
u₂ = -3u₁
The final velocity of both object is represented by v
Applying the principle of momentum conservation
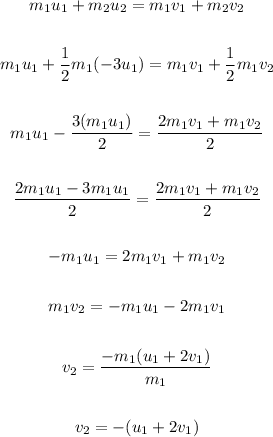
From the last equation, make v₁ the subject of the formula instead