Question

If ABC is an equilateral triangle and BD = 54 inches. find the value of x round to the nearest tenth

Answer 1

Step 1

Draw half of the given triangle

Step 2

State a known fact of an equilateral triangle to help with the question

Since the triangle is an equilateral triangle, each angle in triangle ADC = 60 degrees

Because the sum of angles in a triagle = 180 degrees and an equilateral triangle has all sides and angles equal

Therefore each angle = 180/3 = 60 degrees

so in the triangle ABD,

Step 3

Find the value of x using a trigonometric ratio

To find the length of x, we will use the trig ratio SOH(sine, opposite, hypothenuse)

`\begin{gathered} \text{Sine 60 = }\frac{opposite}{\text{hypothenuse}} \\ \text{opposite}=\text{ 54inches} \\ \text{hypothenuse = x inches} \end{gathered}`

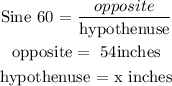
After substitution we will have that

`\begin{gathered} \sin e\text{ 60 = }(54)/(x) \\ \text{but sine 60 = }\frac{\sqrt[]{3}}{2} \\ \frac{\sqrt[]{3}}{2}=(54)/(x) \\ \sqrt[]{3}x=108 \\ x\text{ =}\frac{108}{\sqrt[]{3}} \\ x\text{ =36}\sqrt[]{3} \\ x\text{ }\approx\text{62.4 inches to the nearest tenth} \end{gathered}`

Therefore, x = 62.4 inches to the nearest tenth