Question

A small jet can fly 889 miles in 3.5 hours with a tailwind but only 651 miles in 3.5 hours into a headwind. Find the speed of the jet in still air and the speed of the wind.

Answer 1

Given:

`\begin{gathered} D_{is\tan ace\text{ travelled during tail wind}}=889miles \\ T_{\text{ime taken during tail wind}}=3.5hours \\ D_{is\tan ce\text{ travelled during headwind}}=651miles \\ T_{\text{ime taken during headwind}}=3.5hours \end{gathered}`

To Determine: The speed of the jet in still air and the speed of the wind

Represent the speed of the jet in still air and the speed of the wind with unknowns

`\begin{gathered} T_{he\text{ sp}eed\text{ of the jet in still air}}=x \\ T_{he\text{ sp}ed\text{ of the wind}}=y \end{gathered}`

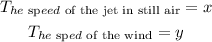
Note that the speed, distance, and time is related by the formula below

`S_{\text{peed}}=\frac{D_(is\tan ce)}{T_{\text{ime}}}`

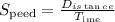
Calculate the speed during the tailwind and the headwind

`S_{\text{peed during tail wind}}=(889)/(3.5)=254milesperhour`
`S_{\text{peed during headwind}}=(651)/(3.5)=186milesperhour`

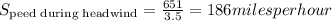
Note that during the tailwild, the speed of the wind and the speed of the jet in still air are in the same direction. Also during the headwind, the speed of the wind and the speed of the jet in still air are in opposite direction. Therefore average speed during the tailwind and the headwind would be

`\begin{gathered} equation1\colon x+y=254 \\ equation2\colon x-y=186 \end{gathered}`

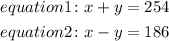
Combine the two equations: Add equation 1 and equation 2 to eliminate y as shown below

`\begin{gathered} x+x-y+y=254+186 \\ 2x=440 \\ x=(440)/(2) \\ x=220\text{ miles per hour} \end{gathered}`

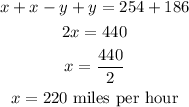
Substitute x = 220 in equation 1

`\begin{gathered} x+y=254 \\ 220+y=254 \\ y=254-220 \\ y=34\text{ miles per hour} \end{gathered}`

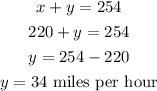
Hence:

The speed of the jet in still air is 220 miles per hour

The speed of the wind is 34 miles per hour