Question

Measurement and conversion (d = rt) Mackenzies bike can go 12 mph. She needs to be at a store at 10:00 A.M.. Of the store is 20 miles away, what time will she need to leave?

Answer 1

We have to use the equation

`d=rt`

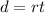
Where r = 12 mph, d = 20 miles, let's find t.

`\begin{gathered} 20=12t \\ t=(20)/(12)=(10)/(6)=(5)/(3) \end{gathered}`

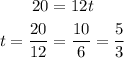
She'll take 5/3 hours to get there which is equivalent to 1 2/3 hours.

Let's transform 2/3 hours to minutes to find the exact time she has to leave.

We know that 1 hour is 60 minutes.

`(2)/(3)hr\cdot(60\min)/(1hr)=(120)/(3)\min =40\min`

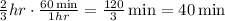
So, she will take exactly 1 hour and 40 minutes.

If you have to get there at 10:00 A.M., then she has to leave at 8:20 A.M.