Question

Read each problem carefully. Solve each quadratic equation for the variable(s) specified. Be sure to show all of your work. Explain in two to three sentences what the meof the solution(s) are in relation to the problem situation.1. An object is propelled off of a platform that is 75 feet high at a speed of 45 feet per second (ft./s). The height of the object off the ground is given by the formulah(t) = - 16t^2 + 45t + 75, where h(t) is the object's height at time (t) seconds after the object is propelled. The downward negative pull on the object is represented by -16t^2? Solvefor t.

Answer 1

`h(t)=-16t^2+45t+75`

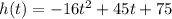
Solve for t using quadratic formula:

`\begin{gathered} t=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ \end{gathered}`

Where:

`\begin{gathered} a=-16 \\ b=45 \\ c=75 \end{gathered}`

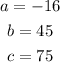
so:

`\begin{gathered} t=\frac{-45\pm\sqrt[]{45^2-4(-16)(75)}}{2(-16)} \\ t=\frac{-45\pm5\sqrt[]{273}}{-32} \\ so\colon \\ t\approx-1.175s \\ or \\ t\approx3.988s \end{gathered}`

We take the positive time, therefore:

Answer:

t = 3.988s

The object will hit the ground after approximately 3.988 seconds.

We can also say that the object will remain in the air for less than 4 seconds.