Question

I already did part a and the magnitude for all the parts. I need help finding the direction.

Answer 1

b)

Given that,

A=10 N at 0°

B=20 N at 180°

As we can see these two vectors are in opposite directions.

Thus the resultant vector will be in the direction of the vector with the higher magnitude.

In vector addition we can write,

`C=10\cos 0^0+20\cos 180^0`

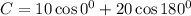
Where C is the resultant vector.

Thus magnitude of R will be equal to,

`R=-10\text{ N}`

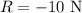
The negative sign indicates that the direction of the C is along the negative x-axis.

Thus the magnitude of C is 10 N and the direction of the C is 180°

a)

Given,

A= 10 N at 0°

B=20 N at 0°

The the vector C is

`C=10\cos 0^0+20\cos 0^0=30\text{ N}`

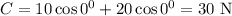
The direction of R will be along the direction of these two vectors, as they are in the same direction.

Thus the magnitude of the vector C will be 30 N and the direction of the vector C is 0°

c)

Given,

A=10 N at 180°

B=20 N at 180°

Thus the vector C is

`C=10\cos 180^0+20\cos 180^0=-30\text{ N}`

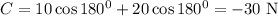
The direction of the vector C will be along the direction of the negative x-axis, as these two vectors are along the negative x-axis.

Thus the magnitude of the vector C is 30 N and the direction of the vector C is 180°

d)

Given,

A= 10 N at 0°

B=20 N at 90°

The x-component of the vector C will be,

`C_x=10\cos 0^0+20\cos 90^0=10\text{ }\hat{\text{i}}`

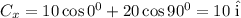
The y- component of C will be,

`C_y=10\sin 0^0+20\sin 90^0=20\text{ }\hat{j}`

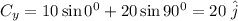
The magnitude of vector C is given by,

`\begin{gathered} C=\sqrt[]{C^2_x+C^2_y}_{} \\ =\sqrt[]{10^2+20^2} \\ =22.36\text{ N} \end{gathered}`

The direction of the vector C will be,

`\begin{gathered} \theta=\tan ^(-1)(C_y)/(C_x) \\ =\tan ^(-1)(20)/(10) \\ =63.43^0 \end{gathered}`

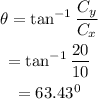
Thus the magnitude of C will be 22.36 N and the direction of C will be 63.43°

e)

Given that

A= 10 N at 90°

B=20 N at 0°

The x-component of the vector C will be

`C_x=10\cos 90^0+20\cos 0^0=20\text{ }\hat{\text{i}}`

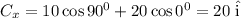
The y-component of the vector C will be

`C_y=10\sin 90^0+20\sin 0^0=10\text{ }\hat{j}`

The magnitude of the vector C will be

`\begin{gathered} C=\sqrt[]{C^2_x+C^2_y}_{} \\ =\sqrt[]{20^2+10^2} \\ =22.36\text{ N} \end{gathered}`

The direction of vector C will be,

`\begin{gathered} \theta=\tan ^(-1)(C_y)/(C_x) \\ =\tan ^(-1)(10)/(20) \\ =26.56^0 \end{gathered}`

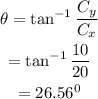
Thus the magnitude of the vector C will be 22.36 N and the direction of the vector C will be 26.56°