As given by the question
There are given that the function
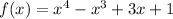
Now,
By the defination, a polynomial of n degree, has a maximum turning points of:

Therefore, if you have the polynomial given in the problem, which is a polynomial of degree 4, that means (n=4).
The maximum number of turns can be obtained as following:

Hence, the maximum number of turns in the graph is 3.