Answer:
The recursive formula is;

Step-by-step explanation:
The recursive formula for geometric progression can be written as;
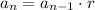
where r is the common difference.
Let us find the common difference of the given sequence;
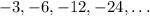
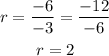
we can now substitute the value of r into the recursive formula;

Therefore, the recursive formula is;
